Page 204 - Maxwell House

184 Chapter 4

Let us pull (4.69) into (3.72) to check the fulfillment of radiation condition (3.72) and
to be sure that we have gotten the unique solution

∆ 2
2
2

lim( ||) = � � sin [W] (4.70)
0
→∞ 2√2
Evidently, the value on the right-hand side of (4.70) is proportional to the power (look
at the unit dimension) radiated by the dipole and equal to some constant being
independent of the radial coordinate. Therefore, the vector potential (4.61) and all the
following expressions are the unique solution of Maxwell’s equations and define the
EM fields correctly.
6. Let us consider an arbitrary sphere with the surface extending up to far-field zone and is
centered at the dipole. Then integrating (4.69) over such big sphere, we can find using
(3.50) the total active radiated power

2
∆ 2 1
3
2
= ∯ ∘ = � 2√2 � ∫ 0 ∫ 0 2 sin (4.71)
Σ
0

Here = sin (see Appendix). Evaluating the inner integral as
2
0
1
∫ sin = − ∫ sin (cos) = ∫ (1 − ) = 4 3 we have

2
2
3
⁄
0 0 −1
2 2 2
) (4.72)
8 ∆ ∆ 2 ∆ 2
= � � = � � = 40 � � (
Σ 0 0
3 2√2 3
7. According to (4.72), there are two choices how to deliver or receive the particular level of
power: excite high current or make the dipole lengthier. Evidently, the second choice
is superior because higher current leads to greater Ohmic loss proportional to the square of
the current magnitude in dipole. We will show later that up to ∆/ ≤ 1 the electric dipole
like shown in Figure 4.2.1b keeps its pattern diagram almost unaltered. That is why such
simplest radiator is one of the most broadly used broadband antenna.
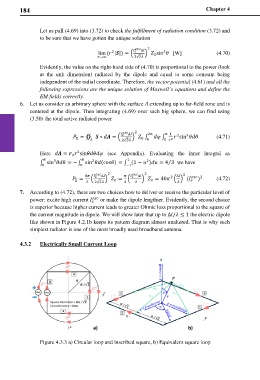
4.3.2 Electrically Small Current Loop

Figure 4.3.3 a) Circular loop and inscribed square, b) Equivalent square loop