Page 318 - Maxwell House

298 Chapter 6

wave as the wave superposition of incident and reflected from the curved inside and outer
conductive surfaces as it is demonstrated in Figure 6.4.2a, d.

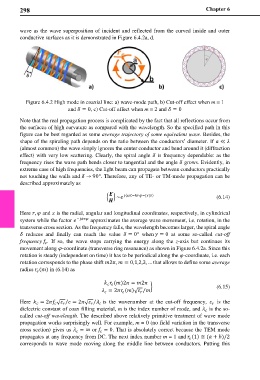
Figure 6.4.2 High mode in coaxial line: a) wave-mode path, b) Cut-off effect when m = 1
and = 0, c) Cut-off effect when m = 2 and = 0
Note that the real propagation process is complicated by the fact that all reflections occur from
the surfaces of high curvature as compared with the wavelength. So the specified path in this
figure can be best regarded as some average trajectory of some equivalent wave. Besides, the
shape of the spiraling path depends on the ratio between the conductors’ diameter. If ≪
(almost common) the wave simply ignores the center conductor and bend around it (diffraction
effect) with very low scattering. Clearly, the spiral angle is frequency dependable: as the
frequency rises the wave path bends closer to tangential and the angle grows. Evidently, in
extreme case of high frequencies, the light beam can propagate between conductors practically
not touching the walls and → 90°. Therefore, any of TE- or TM-mode propagation can be
described approximately as

� � ~ (−−||) (6.14)

Here , and is the radial, angular and longitudinal coordinates, respectively, in cylindrical
system while the factor − approximates the average wave movement, i.e. rotation, in the
transverse cross section. As the frequency falls, the wavelength becomes larger, the spiral angle
reduces and finally can reach the value = 0° when = 0 at some so-called cut-off
frequency . If so, the wave stops carrying the energy along the z-axis but continues its

movement along -coordinate (transverse ring resonance) as shown in Figure 6.4.2a. Since this
rotation is steady (independent on time) it has to be periodical along the -coordinate, i.e. each
rotation corresponds to the phase shift 2, = 0,1,2,3, … that allows to define some average
radius () in (6.14) as

()2 = 2

� (6.15)
= 2 () √
⁄

Here = 2 = 2 √ is the wavenumber at the cut-off frequency, is the
⁄
⁄
√
dielectric constant of coax filling material, m is the index number of mode, and is the so-

called cut-off wavelength. The described above relatively primitive treatment of wave mode
propagation works surprisingly well. For example, m = 0 (no field variation in the transverse
cross section) gives us = ∞ or = 0. That is absolutely correct because the TEM mode

propagates at any frequency from DC. The next index number m = 1 and (1) ≅ ( + )/2

corresponds to wave mode moving along the middle line between conductors. Putting this