Page 323 - Maxwell House

FEED LINE BASICS 303

or even silver with extra top thin-film layer of gold to prevent corrosion. The main problem of
such approach is a big difference in thermal expansion of common dielectrics and metals. It
means that broad temperature fluctuations may be a cause of the metal layer detachment.

Note in conclusion that the described E-field pattern corresponds to the class of TE-modes. The
total spectrum of wave modes is infinite, consists of TE- and TE-modes and can be found
analyzing the wave incident on and reflecting from all four metal walls. However, in this case,
the straight wave equation solutions become more preferable.

Figure 6.4.1f demonstrates a flexible WR that looks like an accordion and can be bent the same
way. It is typically used as short sections connecting some system elements that, for example,
move about each other or cannot be connected by straight rigid WR because of installation
needs. They can help ease the adverse effects of thermal expansion and vibration. In general,
flexible WRs are jacketed in plastic skin providing protection against mechanical damages and
corrosion. Flexible WR specification typically includes a minimum curve radius. More bending
may lead to permanent damage of WR. The theoretical analysis of corrugated WRs is a quite
challenging mathematical task and, in general, provided numerically. But some simple
conclusions can be drawn without it. For example, the attenuation must be higher since the
longitudinal electric current travels longer path along a curved surface than in regular WR. The
power handling drops because of heightened electrical field intensity around the narrow grooves
leading to corona and breakdown between them. We may also expect that new complicated
field pattern is more frequency dependable. Therefore, flexible WRs typically are more
dispersive depending on corrugation softness and propagating mode structure.

6.4.4 Waveguide Circular (WC)
Figure 6.4.1g demonstrates WC that carries electromagnetic waves practically the same way as
WR. As before, there is no chance for TEM-mode propagation. Essentially, everything we have
told about the WR can be applied to WC. Particularly, the dominant TE-modes of WC and WR
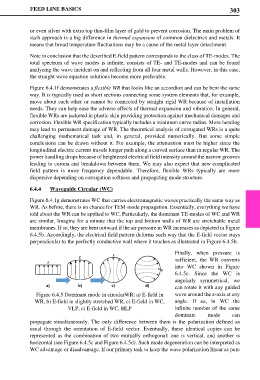
are similar. Imagine for a minute that the top and bottom walls of WR are stretchable metal
membranes. If so, they are bent outward if the air pressure in WR increases as depicted in Figure
6.4.5b. Accordingly, the electrical field pattern deforms such way that the E-field vector stays
perpendicular to the perfectly conductive wall where it touches as illustrated in Figure 6.4.5b.
Finally, when pressure is
E
E sufficient, the WR converts
E
into WC shown in Figure
E
6.4.5c. Since the WC is
angularly symmetrical, we
a) b) c) d) can rotate it with any guided
Figure 6.4.5 Dominant mode in circularWR: a) E-field in wave around the z-axis at any
WR, b) E-field in slightly stretched WR, c) E-field in WC, angle. If so, in WC the
VLP, c) E-field in WC, HLP infinite number of the same
dominant mode can
propagate simultaneously. The only difference between them is the polarization defined as
usual through the orientation of E-field vector. Eventually, these identical copies can be
represented as the combination of two mutually orthogonal: one is vertical, and another is
horizontal (see Figure 6.4.5c and Figure 6.4.5d). Such mode degeneration can be interpreted as
WC advantage or disadvantage. If our primary task to keep the wave polarization linear as pure