Page 321 - Maxwell House

FEED LINE BASICS 301

= sin (6.19)

while it forms the standing wave pattern with zero E-fields on a metal surface, i.e. x = 0, as
required. We know that the function sin (cos) is periodic function that has the infinite
number of zeros cos = , = 1,2, …. If so, we can put the second vertical metal wall,
as shown in Figure 6.4.3b, and do not break the boundary condition = 0 if

cos = ()⁄ = 2 (6.20)
⁄
Then the propagation constant is equal to

= �1 − cos = �1 − ( 2) (6.21)
2
2
⁄
Figure 6.4.3c demonstrates the standing wave distribution of E-field between the planes for m
= 1. Now we are ready to take the last step and close the metal surface putting additional two
metal planes as shown in Figure 6.4.3d. That is permitted because they are perpendicular to the
vector of the electrical field and do not break any boundary condition from Table 2.2. If so, we
get the new type of line, an entirely shielded WR. The red color reveals areas with the peak E-
field intensity. It is a remarkable fact that Maxwell’s equations in combination with boundary
conditions “dictate” the WR what kind of partial wave to choose for propagation.
The dominant mode corresponds to m = 1 and evidently, stops propagating when = 0 (see
Figure 6.4.4a) and = 0 at the cut-off wavelength 1 = 2 according to (6.21). The next
higher mode corresponding to m = 2 has 2 = . Therefore, the single mode regime when the
total EM energy caries by only one dominant wave mode takes place if < < or
2 1
/2 < < (6.22)

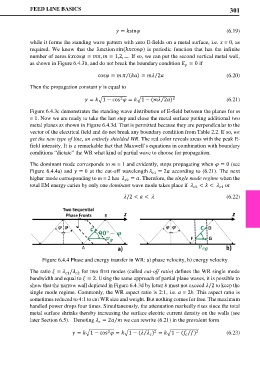
Figure 6.4.4 Phase and energy transfer in WR: a) phase velocity, b) energy velocity
The ratio = / for two first modes (called cut-off ratio) defines the WR single mode
2
1
bandwidth and equal to = 2. Using the same approach of partial plane waves, it is possible to
show that the narrow wall depicted in Figure 6.4.3d by letter b must not exceed /2 to keep the
single mode regime. Commonly, the WR aspect ratio is 2:1, i.e. a = 2b. This aspect ratio is
sometimes reduced to 4:1 to cut WR size and weight. But nothing comes for free. The maximum
handled power drops four times. Simultaneously, the attenuation markedly rises since the total
metal surface shrinks thereby increasing the surface electric current density on the walls (see
later Section 6.5). Denoting = 2 we can rewrite (6.21) in the prevalent form
⁄

= �1 − cos = �1 − ( ) = �1 − ( ) (6.23)
2
2
2
⁄
⁄