Page 320 - Maxwell House

300 Chapter 6

Coaxial cable applications are typical for frequencies below 3 GHz and up to 300 GHz as short
sections. The attenuation might be around 3 dB per 100 m at 100 MHz, increases to 10 dB per
100 m at 1 GHz, and is around 50 dB per100 m at 10 GHz. Their power handling is on the order
of several kilowatts at 100 MHz and drops to 1000 W at 2 GHz, being limited primarily by high
electric field concentration around inner conductor and dielectric heating.

6.4.3 Waveguide Rectangular (WR)
Simply put, the WRs in Figure 6.4.1e as any other hollow waveguide are highly conductive
metal (copper or aluminum) tubes. Theoretically, the shape of tube cross section can be
arbitrary, but the most commonly used are rectangular, circular and less frequently elliptical.
Evidently, there is no way to connect a two-node DC generator to such WR and circumvent a
short circuit. Therefore, the WRs do not support TEM-mode propagation; the frequency
dispersion is mandatory, and the WRs are relatively narrow-banded. So why would we need
them if they are massive and weighty also? Besides, the technology of circuit components’
integration with WRs is difficult and relatively costly. Nevertheless, they have three significant
advantages: low loss, excellent power handling and the highest level of shielding.
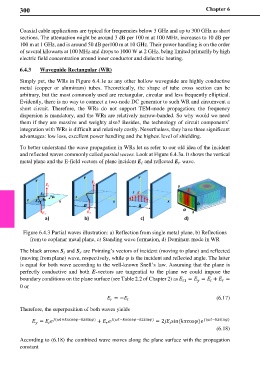
To better understand the wave propagation in WRs let us refer to our old idea of the incident
and reflected waves commonly called partial waves. Look at Figure 6.4.3a. It shows the vertical
metal plane and the E-field vectors of plane incident and reflected wave.

Figure 6.4.3 Partial waves illustration: a) Reflection from single metal plane, b) Reflections
from to coplanar metal plane, c) Standing wave formation, d) Dominant mode in WR

The black arrows and are Pointing’s vectors of incident (moving to plane) and reflected

(moving from plane) wave, respectively, while is the incident and reflected angle. The latter
is equal for both wave according to the well-known Snell’s law. Assuming that the plane is
perfectly conductive and both E-vectors are tangential to the plane we could impose the
boundary conditions on the plane surface (see Table 2.2 of Chapter 2) as 1 = = + =

0 or
= − (6.17)

Therefore, the superposition of both waves yields
= (+cos−sin) + (−cos−sin) = 2 sin (cos) (−sin)

(6.18)
According to (6.18) the combined wave moves along the plane surface with the propagation
constant