Page 326 - Maxwell House

306 Chapter 6

6.5 BASICS OF LINE THEORY

6.5.1 Lumped Circuit Model of a Transmission Line
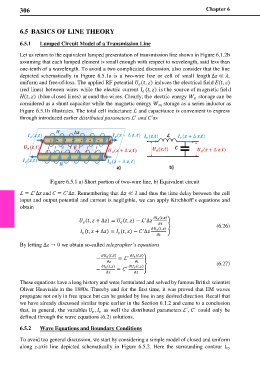
Let us return to the equivalent lumped presentation of transmission line shown in Figure 6.1.2b
assuming that each lumped element is small enough with respect to wavelength, said less than
one-tenth of a wavelength. To avoid a two complicated discussion, also consider that the line
depicted schematically in Figure 6.5.1a is a two-wire line or cell of small length ∆ ≪ ,
uniform and free-of-loss. The applied RF potential (, ) induces the electrical field E(, )

t
(red lines) between wires while the electric current (, ) is he sour ce of magnetic field

li
H(, ) (blue closed nes) around the wires. Clearly, the electric energy storage can be
considered as a shunt capacitor while the magnetic energy storage as a series inductor as

Figure 6.5.1b illustrates. The total cell inductance ℒ and capacitance is convenient to express
through introduced earlier distributed parameters ℒ and as
′
′

Figure 6.5.1 a) Short portion of two-wire line, b) Equivalent circuit

ℒ = ℒ ∆ and = ∆. Remembering that ∆ ≪ and thus the time delay between the cell
′
′
input and output potential and current is negligible, we can apply Kirchhoff’s equations and
obtain
′ (,)
(, + ∆) = (, ) − ℒ ∆

� (6.26)
(,)
′
(, + ∆) = (, ) − ∆

By letting ∆ → 0 we obtain so-called telegrapher’s equations
(,) ′ (,)
− = ℒ
� (6.27)
(,) (,)
− = ′

These equations have a long history and were formulated and solved by famous British scientist
Oliver Heaviside in the 1880s. Thereby and for the first time, it was proved that EM waves
propagate not only in free space but can be guided by line in any desired direction. Recall that
we have already discussed similar topic earlier in the Section 6.1.2 and came to a conclusion
that, in general, the variables , as well the distributed parameters ℒ , could only be
′
′

defined through the wave equations (6.2) solutions.
6.5.2 Wave Equations and Boundary Conditions
To avoid too general discussion, we start by considering a simple model of closed and uniform
along z-axis line depicted schematically in Figure 6.5.2. Here the surrounding contour
0