Page 517 - Mechatronics with Experiments

Printer: Yet to Come
October 9, 2014 8:41 254mm×178mm
JWST499-Cetinkunt
JWST499-c07
ELECTROHYDRAULIC MOTION CONTROL SYSTEMS 503
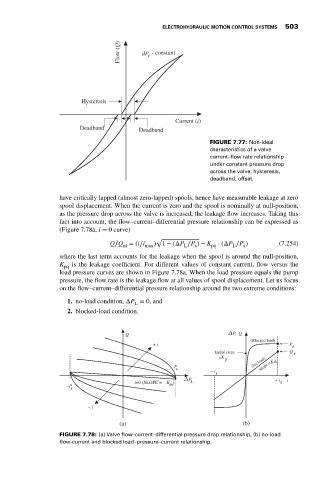
Flow (Q) dP - constant
v

Hysteresis

Current (i)
Deadband Deadband

FIGURE 7.77: Non-ideal
characteristics of a valve
current–flow rate relationship
under constant pressure drop
across the valve: hysteresis,
deadband, offset.

have critically lapped (almost zero-lapped) spools, hence have measurable leakage at zero
spool displacement. When the current is zero and the spool is nominally at null-position,
as the pressure drop across the valve is increased, the leakage flow increases. Taking this
fact into account, the flow–current–differential pressure relationship can be expressed as
(Figure 7.78a, i = 0 curve)
√
Q∕Q = (i∕i ) 1 − (ΔP ∕P ) − K ⋅ (ΔP ∕P ) (7.254)
nl max L s pq L s
where the last term accounts for the leakage when the spool is around the null-position,
K pq is the leakage coefficient. For different values of constant current, flow versus the
load pressure curves are shown in Figure 7.78a. When the load pressure equals the pump
pressure, the flow rate is the leakage flow at all values of spool displacement. Let us focus
on the flow–current–differential pressure relationship around the two extreme conditions:

1. no-load condition, ΔP = 0, and
L
2. blocked-load condition.

Q P, Q
(Blocked load)
x + i P
s
Initial slope Q r
x
=K
p
x P (No load) =K q
s Slope
– i r
x x x x x x
P + i i
i=0 (SLOPE = K ) L r
–P x pq
s
x
– i x
(a) (b)
FIGURE 7.78: (a) Valve flow–current–differential pressure drop relationship, (b) no-load
flow-current and blocked load–pressure–current relationship.