Page 76 - BUKU ARA

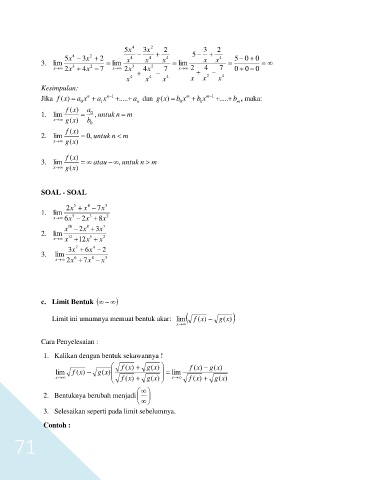
5x 4  3x 2  2  3  2
5x 4  3x 2  2 x 4 x 4 x 4 5 x x 4 5  0  0
3. lim  lim  lim   
x   2x 3  4x 2  7 x   2x 3  4x 2  7 x   2  4  7 0  0  0
x 4 x 4 x 4 x x 2 x 4
Kesimpulan:
m
n
)
)
Jika f ( x  a 0 x  a 1 x n1 .....  a dan g( x  b 0 x  b 1 x m1 .....  b , maka:
n
m
f ( x) a
1. lim  0 , untuk n  m
x  g( x) b 0
f ( x)
0
2. lim  , untuk n  m
x  g( x)
f ( x)

3. lim   atau , untuk n  m
x  g( x)

SOAL - SOAL

2x  x  7x 3
5
4
1. lim
5
3
x  6x  2x  8x 2
10
8
x  2x  3x 7
2. lim
12
5
x  x  12x  x 2
3x  6x  2
7
4
3. lim
4
6
x  2x  7x  x 3

c. Limit Bentuk    
lim
Limit ini umumnya memuat bentuk akar:  f (x )  g (x  )
x 
Cara Penyelesaian :
1. Kalikan dengan bentuk sekawannya !
 f (x )  g (x )  f (x ) g (x )

lim f (x )  g (x )    lim
x   f (x )  g (x )  x  f (x )  g (x )
 
  
2. Bentuknya berubah menjadi 
  
3. Selesaikan seperti pada limit sebelumnya.

Contoh :

71