Page 11 - KIII - TRIGONOMETRIA 3SEC

Trigonometría 3° Secundaria

17
SEMANA

Ángulos cuadrantales.
Entenderemos por ángulo cuadrantal a aquel ángulo en posición normal cuyo lado final coincida con cualquier

semieje del plano cartesiano. La medida de este ángulo siempre tendrá la forma "n " ; n ∈ Z ó "n. 90º".
2

Ejemplo:
Para diferentes valores enteros de "n" tendríamos: n = -3; -2; -1; 0; 1; 2; 3; 4; ….
n . 90 = -270º; -180º; -90º; 0; 90º; 180º; 270º; 360º;

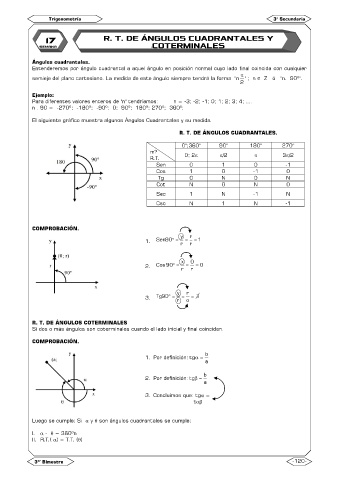
El siguiente gráfico muestra algunos Ángulos Cuadrantales y su medida.

R. T. DE ÁNGULOS CUADRANTALES.

0°;360° 90° 180° 270°
m? 0; 2 /2 3/2
R.T. 
Sen 0 1 0 -1
Cos 1 0 -1 0
Tg 0 N 0 N
Cot N 0 N 0
Sec 1 N -1 N
Csc N 1 N -1

COMPROBACIÓN.
y r
1. Sen90 = r = r = 1

x 0
2. Cos90 = r = r = 0

y r
3. Tg90 = r = o = 

R. T. DE ÁNGULOS COTERMINALES
Si dos o más ángulos son coterminales cuando el lado inicial y final coinciden.

COMPROBACIÓN.

b
1. Por definición: tg =
a

b
2. Por definición: tg =
a

3. Concluimos que: tg =
t

Luego se cumple: Si  y  son ángulos cuadrantales se cumple:

I.  -  = 360ºn
II. R.T.( ) = T.T. ()

er
3 Bimestre -120-