Page 72 - u4

P1: OSO/OVY
GO01962-Smith-v1.cls
UAE-Math-Grade-12-Vol-1-SE-718383-ch4
CHAPTER 4 • •
Applications of Differentiation
262 P2: OSO/OVY QC: OSO/OVY T1: OSO October 25, 2018 17:24 4-52
y
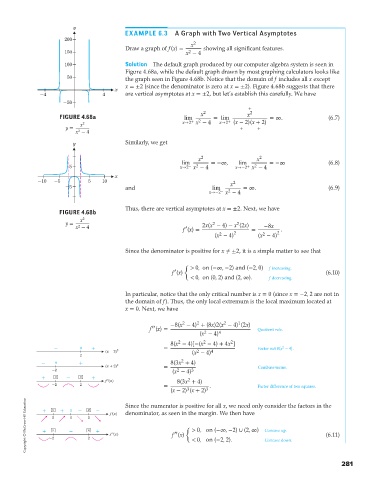
EXAMPLE 6.3 A Graph with Two Vertical Asymptotes
200 2
Draw a graph of f(x) = x showing all significant features.
2
150 x − 4
100 Solution The default graph produced by our computer algebra system is seen in
Figure 4.68a, while the default graph drawn by most graphing calculators looks like
50 the graph seen in Figure 4.68b. Notice that the domain of f includes all x except
x =±2 (since the denominator is zero at x =±2). Figure 4.68b suggests that there
x
-4 4 are vertical asymptotes at x =±2, but let’s establish this carefully. We have
-50
+
x 2 x 2
FIGURE 4.68a x→2 x − 4 = lim + =∞. (6.7)
lim
2
x→2 (x − 2)(x + 2)
+
y = x 2 + +
2
x − 4
y Similarly, we get
lim x 2 = −∞, lim x 2 = −∞ (6.8)
2
2
5 x→2 x − 4 x→−2 x − 4
+
−
x
-10 -5 5 10 x 2
-5 and lim =∞. (6.9)
2
−
x→−2 x − 4
Thus, there are vertical asymptotes at x =±2. Next, we have
FIGURE 4.68b
y = x 2 2 2
2
x − 4 f (x) = 2x(x − 4) − x (2x) = −8x .
′
2
2
(x − 4) 2 (x − 4) 2
Since the denominator is positive for x ≠ ±2, it is a simple matter to see that
{ > 0, on (−∞, −2) and (−2, 0) f increasing.
′
f (x) (6.10)
< 0, on (0, 2) and (2, ∞). f decreasing.
In particular, notice that the only critical number is x = 0 (since x =−2, 2 are not in
the domain of f). Thus, the only local extremum is the local maximum located at
x = 0. Next, we have

2
2
2
1
′′
f (x) = −8(x − 4) + (8x)2(x − 4) (2x) Quotient rule.
2
(x − 4) 4
2
2
2
8(x − 4)[−(x − 4) + 4x ]
2
- 0 + = Factor out 8(x − 4).
2
(x - 2) 3 (x − 4) 4
2
2
- 0 + 8(3x + 4)
(x + 2) 3 = Combine terms.
2
-2 (x − 4) 3
+ × - × +
2
f��(x) 8(3x + 4)
-2 2 = . Factor difference of two squares.
3
(x − 2) (x + 2) 3
Copyright © McGraw-Hill Education + -2 × × + - 0 0 - × 2 2 - f�(x) denominator, as seen in the margin. We then have Concave up. (6.11)
Since the numerator is positive for all x, we need only consider the factors in the
> 0, on (−∞, −2) ∪ (2, ∞)
{
×
+
+
′′
f (x)
f��(x)
-2
< 0, on (−2, 2).
Concave down.
281