Page 74 - u4

T1: OSO
P2: OSO/OVY
QC: OSO/OVY
P1: OSO/OVY
July 4, 2016
GO01962-Smith-v1.cls
UAE_Math_Grade_12_Vol_1_SE_718383_ch3
information, we have 13:38
′
−2
3
2
2
f (x) =−(x + 3x + 3x + 3) (3x + 6x + 3)
[ (x + 1) 2 ]
=−3
3
(x + 3x + 3x + 3) 2
2
( ) 2
=−3 x + 1
2
3
x + 3x + 3x + 3
< 0, for x ≠ a or −1 (6.16)
′
and f (−1) = 0. Thus, f is decreasing for x < a and x > a. Also, notice that the only
critical number is x =−1, but since f is decreasing everywhere except at x = a,
there are no local extrema. Turning to the second derivative, we get
2
3
2
( x + 1 ) 1(x + 3x + 3x + 3) − (x + 1)(3x + 6x + 3)
′′
f (x) =−6
x + 3x + 3x + 3 (x + 3x + 3x + 3) 2
3
2
3
2
−6(x + 1)
2
3
= (−2x − 6x − 6x)
2
(x + 3x + 3x + 3) 3
3
2
12x(x + 1)(x + 3x + 3)
= .
2
(x + 3x + 3x + 3) 3
3
2
Since (x + 3x + 3) > 0 for all x (why is that?), we need not consider this factor.
Considering the remaining factors, we have the number lines shown here.
- 0 +
12x
0
- 0 +
(x + 1)
-1
- 0 +
3
2
(x + 3x + 3x + 3)
a = -2.2599…
- × + 0 - 0 +
f��(x)
a -1 0
Thus, we have that
{ > 0, on (a, −1) and (0, ∞) Concave up.
′′
f (x) (6.17)
< 0, on (−∞,a) and (−1, 0). Concave down.
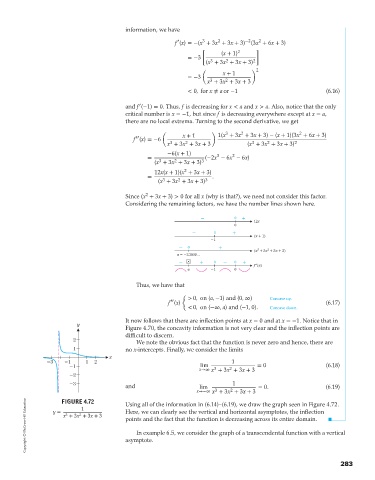
It now follows that there are inflection points at x = 0 and at x =−1. Notice that in
y
Figure 4.70, the concavity information is not very clear and the inflection points are
difficult to discern.
2 We note the obvious fact that the function is never zero and hence, there are
1 no x-intercepts. Finally, we consider the limits
x
-3 -1 1 2 1
-1 lim = 0 (6.18)
2
x→∞ x + 3x + 3x + 3
3
-2
-3 1
and lim = 0. (6.19)
3
x→−∞ x + 3x + 3x + 3
2
Copyright © McGraw-Hill Education y = x + 3x + 3x + 3 Here, we can clearly see the vertical and horizontal asymptotes, the inflection
FIGURE 4.72
Using all of the information in (6.14)–(6.19), we draw the graph seen in Figure 4.72.
1
3
2
points and the fact that the function is decreasing across its entire domain.
In example 6.5, we consider the graph of a transcendental function with a vertical
asymptote.
283