Page 73 - u4

P2: OSO/OVY
P1: OSO/OVY
GO01962-Smith-v1.cls
UAE_Math_Grade_12_Vol_1_SE_718383_ch3
y QC: OSO/OVY T1: OSO July 4, 2016 13:38
However, since x = 2, −2 are not in the domain of f, there are no inflection points.
It is an easy exercise to verify that
6
4 x 2
lim = 1 (6.12)
2 x→∞ x − 4
2
x
-6 -4 4 6
-2
-4 and lim 2 x 2 = 1. (6.13)
-6 x→−∞ x − 4
From (6.12) and (6.13), we have that y = 1 is a horizontal asymptote, both as x → ∞
FIGURE 4.69 and as x → −∞. Finally, we observe that the only x-intercept is at x = 0. We
x 2
y = summarize the information in (6.7)–(6.13) in the graph seen in Figure 4.69.
x − 4
2
In example 6.4, we need to use computer-generated graphs, as well as a rootfinding
method to determine the behavior of the function.

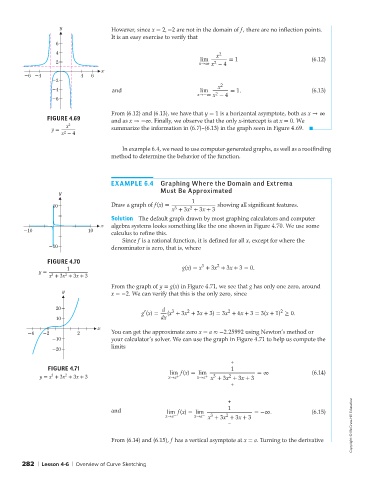
EXAMPLE 6.4 Graphing Where the Domain and Extrema
y Must Be Approximated
1
10 Draw a graph of f(x) = showing all significant features.
2
x + 3x + 3x + 3
3
Solution The default graph drawn by most graphing calculators and computer
x algebra systems looks something like the one shown in Figure 4.70. We use some
-10 10 calculus to refine this.
Since f is a rational function, it is defined for all x, except for where the
-10 denominator is zero, that is, where

FIGURE 4.70
2
3
y = 1 g(x) = x + 3x + 3x + 3 = 0.
x + 3x + 3x + 3
3
2
From the graph of y = g(x) in Figure 4.71, we see that g has only one zero, around
y x =−2. We can verify that this is the only zero, since
20 d
′
3
2
2
2
g (x) = (x + 3x + 3x + 3) = 3x + 6x + 3 = 3(x + 1) ≥ 0.
10 dx
x
-4 -2 2 You can get the approximate zero x = a ≈−2.25992 using Newton’s method or
-10 your calculator’s solver. We can use the graph in Figure 4.71 to help us compute the
limits
-20
+
FIGURE 4.71 1
lim f(x) = lim
2
3
3
2
+
y = x + 3x + 3x + 3 x→a + x→a x + 3x + 3x + 3 =∞ (6.14)
+
+
and lim f(x) = lim 1 = −∞. (6.15)
3
2
x→a − x→a − x + 3x + 3x + 3
− Copyright © McGraw-Hill Education
From (6.14) and (6.15), f has a vertical asymptote at x = a. Turning to the derivative

282 | Lesson 4-6 | Overview of Curve Sketching