Page 84 - Linear Models for the Prediction of Animal Breeding Values 3rd Edition

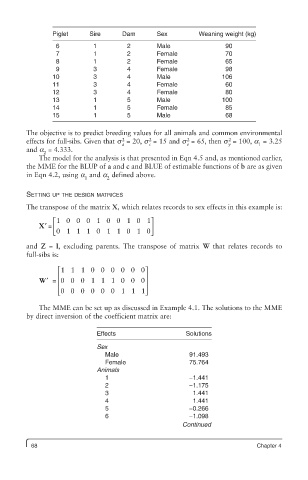
Piglet Sire Dam Sex Weaning weight (kg)
6 1 2 Male 90
7 1 2 Female 70
8 1 2 Female 65
9 3 4 Female 98
10 3 4 Male 106
11 3 4 Female 60
12 3 4 Female 80
13 1 5 Male 100
14 1 5 Female 85
15 1 5 Male 68

The objective is to predict breeding values for all animals and common environmental
2
2
2
2
effects for full-sibs. Given that s = 20, s = 15 and s = 65, then s = 100, a = 3.25
a c e y 1
and a = 4.333.
2
The model for the analysis is that presented in Eqn 4.5 and, as mentioned earlier,
the MME for the BLUP of a and c and BLUE of estimable functions of b are as given
in Eqn 4.2, using a and a defined above.
1 2
SETTING UP THE DESIGN MATRICES
The transpose of the matrix X, which relates records to sex effects in this example is:
⎡1 000 1 0 0 1 01 ⎤
X ′ = ⎢ ⎥
⎣ 0 1 1 1 0 1 10 10 ⎦
and Z = I, excluding parents. The transpose of matrix W that relates records to
full-sibs is:

⎡111 000000 ⎤
⎢ ⎥
W ′ = 000 111 000 ⎥
⎢
⎣ ⎢000000 111 ⎥ ⎦

The MME can be set up as discussed in Example 4.1. The solutions to the MME
by direct inversion of the coefficient matrix are:

Effects Solutions

Sex
Male 91.493
Female 75.764
Animals
1 −1.441
2 −1.175
3 1.441
4 1.441
5 −0.266
6 −1.098
Continued

68 Chapter 4