Page 117 - Microeconomics, Fourth Edition

c03consumerpreferencesandtheconceptofutility.qxd 6/14/10 2:54 PM Page 91

3.2 UTILITY FUNCTIONS 91
Can the indifference curve U 1 intersect either axis? Note that both MU x and MU y are positive when-
Since U 1 is positive, x and y must both be positive (assum- ever the consumer has positive amounts of x and y.
ing the consumer is buying positive amounts of both Therefore, indifference curves will be negatively sloped.
goods). If U 1 intersected the x axis, the value of y at that This means that as the consumer increases x along an in-
point would be zero; similarly, if U 1 intersected the y axis, difference curve, y must decrease. Since MRS x,y
the value of x at that point would be zero. If either x or y MU x MU y y x , as we move along the indifference
were zero, the value of U 1 would also be zero, not 128. curve by increasing x and decreasing y, MRS x,y y x
Therefore, the indifference curve U 1 cannot intersect will decrease. So MRS x,y depends on x and y, and we
either axis. have diminishing marginal rate of substitution of x for y.
Is MRS x,y diminishing for U 1 ? Figure 3.11 shows
Similar Problems: 3.10, 3.11
that U 1 is bowed in toward the origin; therefore, MRS x,y
is diminishing for U 1 .
(b) Figure 3.11 also shows the indifference curve
U 2 200, which lies up and to the right of U 1 128.

Learning-By-Doing Exercise 3.4 involves indifference curves with an increasing
marginal rate of substitution. Such curves are theoretically possible but not usually
encountered.

LEARNING-BY-DOING EXERCISE 3.4
S
D
E
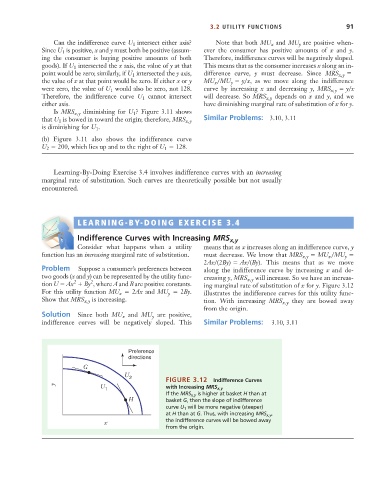
Indifference Curves with Increasing MRS x,y
Consider what happens when a utility means that as x increases along an indifference curve, y
function has an increasing marginal rate of substitution. must decrease. We know that MRS x,y MU x MU y
2Ax (2By) Ax (By) . This means that as we move
Problem Suppose a consumer’s preferences between along the indifference curve by increasing x and de-
two goods (x and y) can be represented by the utility func- creasing y, MRS x,y will increase. So we have an increas-
2
2
tion U Ax By , where A and B are positive constants. ing marginal rate of substitution of x for y. Figure 3.12
For this utility function MU x 2Ax and MU y 2By. illustrates the indifference curves for this utility func-
Show that MRS x,y is increasing. tion. With increasing MRS x,y they are bowed away
from the origin.
Solution Since both MU x and MU y are positive,
indifference curves will be negatively sloped. This Similar Problems: 3.10, 3.11

Preference
directions
G
U
2 FIGURE 3.12 Indifference Curves
U
y
1 with Increasing MRS x,y
If the MRS x,y is higher at basket H than at
H basket G, then the slope of indifference
curve U 1 will be more negative (steeper)
at H than at G. Thus, with increasing MRS x,y ,
x the indifference curves will be bowed away
from the origin.