Page 127 - Fiber Optic Communications Fund

108 Fiber Optic Communications

3.4 Laser Examples
3.4.1 Ruby Laser

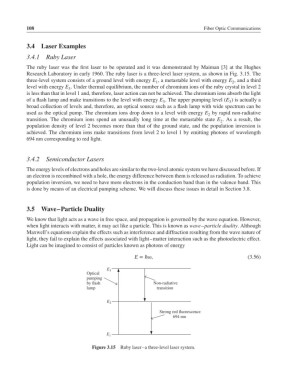
The ruby laser was the first laser to be operated and it was demonstrated by Maiman [3] at the Hughes
Research Laboratory in early 1960. The ruby laser is a three-level laser system, as shown in Fig. 3.15. The
three-level system consists of a ground level with energy E , a metastable level with energy E , and a third
1 2
level with energy E . Under thermal equilibrium, the number of chromium ions of the ruby crystal in level 2
3
is less than that in level 1 and, therefore, laser action can not be achieved. The chromium ions absorb the light
of a flash lamp and make transitions to the level with energy E . The upper pumping level (E ) is actually a
3
3
broad collection of levels and, therefore, an optical source such as a flash lamp with wide spectrum can be
used as the optical pump. The chromium ions drop down to a level with energy E by rapid non-radiative
2
transition. The chromium ions spend an unusually long time at the metastable state E . As a result, the
2
population density of level 2 becomes more than that of the ground state, and the population inversion is
achieved. The chromium ions make transitions from level 2 to level 1 by emitting photons of wavelength
694 nm corresponding to red light.

3.4.2 Semiconductor Lasers
The energy levels of electrons and holes are similar to the two-level atomic system we have discussed before. If
an electron is recombined with a hole, the energy difference between them is released as radiation. To achieve
population inversion, we need to have more electrons in the conduction band than in the valence band. This
is done by means of an electrical pumping scheme. We will discuss these issues in detail in Section 3.8.

3.5 Wave–Particle Duality

We know that light acts as a wave in free space, and propagation is governed by the wave equation. However,
when light interacts with matter, it may act like a particle. This is known as wave–particle duality. Although
Maxwell’s equations explain the effects such as interference and diffraction resulting from the wave nature of
light, they fail to explain the effects associated with light–matter interaction such as the photoelectric effect.
Light can be imagined to consist of particles known as photons of energy
E = ℏ, (3.56)

E 3
Optical
pumping
by flash Non-radiative
lamp transition

E 2
Strong red fluorescence
694 nm

E 1

Figure 3.15 Ruby laser–a three-level laser system.