Page 122 - Fiber Optic Communications Fund

Lasers 103

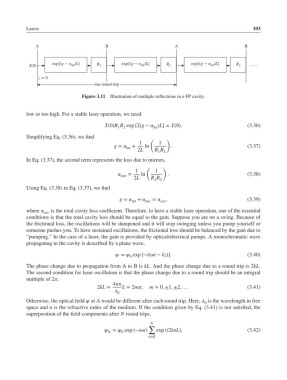
A B A B

exp[(g − α int )L] exp[(g − α int )L] exp[(g − α int )L]
I(0) R 2 R 1 R 2 ... ...
z = 0
one round trip

Figure 3.11 Illustration of multiple reflections in a FP cavity.

low or too high. For a stable laser operation, we need
(0)R R exp [2(g − )L]= (0). (3.36)
1 2
int
Simplifying Eq. (3.36), we find
( )
1 1
g = int + ln . (3.37)
2L R R
1 2
In Eq. (3.37), the second term represents the loss due to mirrors,
( )
1 1
= ln . (3.38)
mir
2L R R
1 2
Using Eq. (3.38) in Eq. (3.37), we find
g = int + mir = cav , (3.39)

where is the total cavity loss coefficient. Therefore, to have a stable laser operation, one of the essential
cav
conditions is that the total cavity loss should be equal to the gain. Suppose you are on a swing. Because of
the frictional loss, the oscillations will be dampened and it will stop swinging unless you pump yourself or
someone pushes you. To have sustained oscillations, the frictional loss should be balanced by the gain due to
“pumping.” In the case of a laser, the gain is provided by optical/electrical pumps. A monochromatic wave
propagating in the cavity is described by a plane wave,

= exp [−i(t − kz)]. (3.40)
0
The phase change due to propagation from A to B is kL. And the phase change due to a round trip is 2kL.
The second condition for laser oscillation is that the phase change due to a round trip should be an integral
multiple of 2,
4n
2kL = L = 2m, m = 0, ±1, ±2, … (3.41)
0
Otherwise, the optical field at A would be different after each round trip. Here, is the wavelength in free
0
space and n is the refractive index of the medium. If the condition given by Eq. (3.41) is not satisfied, the
superposition of the field components after N round trips,
N
∑
= exp (−it) exp (i2knL), (3.42)
0
N
n=0