Page 125 - Fiber Optic Communications Fund

106 Fiber Optic Communications

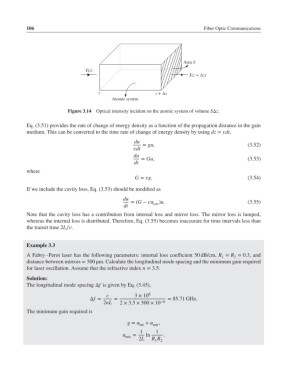
Area S
I(z)
I(z + ∆z)

z z + ∆z
Atomic system

Figure 3.14 Optical intensity incident on the atomic system of volume SΔz.

Eq. (3.51) provides the rate of change of energy density as a function of the propagation distance in the gain
medium. This can be converted to the time rate of change of energy density by using dz = dt,
du
= gu, (3.52)
dt
du
= Gu, (3.53)
dt
where
G = g. (3.54)

If we include the cavity loss, Eq. (3.53) should be modified as
du
=(G − cav )u. (3.55)
dt
Note that the cavity loss has a contribution from internal loss and mirror loss. The mirror loss is lumped,
whereas the internal loss is distributed. Therefore, Eq. (3.55) becomes inaccurate for time intervals less than
the transit time 2L∕.

Example 3.3
A Fabry–Perot laser has the following parameters: internal loss coefficient 50 dB/cm, R = R = 0.3, and
1 2
distance between mirrors = 500 μm. Calculate the longitudinal mode spacing and the minimum gain required
for laser oscillation. Assume that the refractive index n = 3.5.
Solution:
The longitudinal mode spacing Δf is given by Eq. (3.45),
c 3 × 10 8
Δf = = = 85.71 GHz.
2nL 2 × 3.5 × 500 × 10 −6
The minimum gain required is

g = + ,
int mir
1 1
= ln .
mir
2L R R
1 2