Page 132 - Fiber Optic Communications Fund

Lasers 113

of the ground state is negligibly small compared with the population density of the excited state [10, 11].
Assuming N ≈ 0, Eqs. (3.86) and (3.85) become
1
dN N
2 2
= R pump − GN − , (3.89)
ph
dt 21
dN N
ph ph
= GN + AN − , (3.90)
2
ph
dt ph
where
G = BN ℏ. (3.91)
2
The equations describing the population densities of electrons and photons in a semiconductor laser are
similar to Eqs. (3.89) and (3.90). In Section 3.8, we will solve Eqs. (3.89) and (3.90) for a specific pump-
ing scheme.

3.7 Review of Semiconductor Physics
In conductors, such as metals, electrons move around freely in the lattice and are available for conduction.
These electrons can be drifted by applying an electric field across its terminals. In contrast, the insulators
hardly have free electrons in the lattice and, therefore, they do not conduct. Semiconductor materials have
conduction properties that are typically intermediate between that of a metal and an insulator. For example,
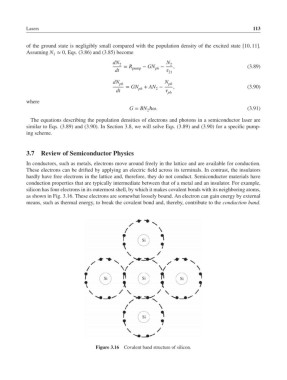
silicon has four electrons in its outermost shell, by which it makes covalent bonds with its neighboring atoms,
as shown in Fig. 3.16. These electrons are somewhat loosely bound. An electron can gain energy by external
means, such as thermal energy, to break the covalent bond and, thereby, contribute to the conduction band.

Si

Si Si Si

Si

Figure 3.16 Covalent band structure of silicon.