Page 133 - Fiber Optic Communications Fund

114 Fiber Optic Communications

Loosely speaking, if an electron is confined to the outermost shell of the atom, it is said to be in the valence
band and if it is moving freely in the lattice, it is said to be in the conduction band. Strictly speaking, atoms of
solid-state materials have such a strong interaction that they cannot be treated as individual entities. Valence
electrons are not attached to individual atoms, instead, they belong to the system of atoms as a whole.
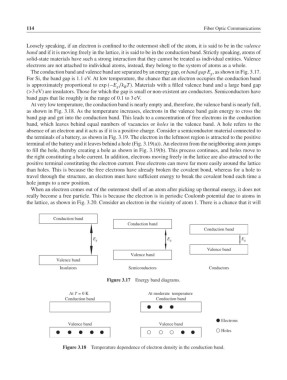
The conduction band and valence band are separated by an energy gap, or band gap E , as shown in Fig. 3.17.
g
For Si, the band gap is 1.1 eV. At low temperature, the chance that an electron occupies the conduction band
is approximately proportional to exp (−E ∕k T). Materials with a filled valence band and a large band gap
g
B
(>3 eV) are insulators. Those for which the gap is small or non-existent are conductors. Semiconductors have
band gaps that lie roughly in the range of 0.1to3 eV.
At very low temperature, the conduction band is nearly empty and, therefore, the valence band is nearly full,
as shown in Fig. 3.18. As the temperature increases, electrons in the valence band gain energy to cross the
band gap and get into the conduction band. This leads to a concentration of free electrons in the conduction
band, which leaves behind equal numbers of vacancies or holes in the valence band. A hole refers to the
absence of an electron and it acts as if it is a positive charge. Consider a semiconductor material connected to
the terminals of a battery, as shown in Fig. 3.19. The electron in the leftmost region is attracted to the positive
terminal of the battery and it leaves behind a hole (Fig. 3.19(a)). An electron from the neighboring atom jumps
to fill the hole, thereby creating a hole as shown in Fig. 3.19(b). This process continues, and holes move to
the right constituting a hole current. In addition, electrons moving freely in the lattice are also attracted to the
positive terminal constituting the electron current. Free electrons can move far more easily around the lattice
than holes. This is because the free electrons have already broken the covalent bond, whereas for a hole to
travel through the structure, an electron must have sufficient energy to break the covalent bond each time a
hole jumps to a new position.
When an electron comes out of the outermost shell of an atom after picking up thermal energy, it does not
really become a free particle. This is because the electron is in periodic Coulomb potential due to atoms in
the lattice, as shown in Fig. 3.20. Consider an electron in the vicinity of atom 1. There is a chance that it will

Conduction band
Conduction band
Conduction band
E g E g E g

Valence band
Valence band
Valence band
Insulators Semiconductors Conductors

Figure 3.17 Energy band diagrams.

At T = 0 K At moderate temperature
Conduction band Conduction band

Electrons
Valence band Valence band
Holes

Figure 3.18 Temperature dependence of electron density in the conduction band.