Page 57 - Fiber Optic Communications Fund

38 Fiber Optic Communications

Jacket Jacket
Cladding Cladding
i max
i Core Core
i
Jacket Jacket
(a) (b)

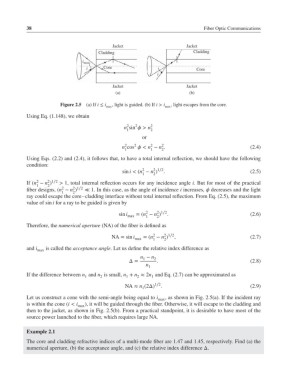
Figure 2.5 (a) If i ≤ i max , light is guided. (b) If i > i max , light escapes from the core.
Using Eq. (1.148), we obtain
2
2
n sin > n 2
1 2
or
2
2
2
2
n cos < n − n . (2.4)
1 1 2
Using Eqs. (2.2) and (2.4), it follows that, to have a total internal reflection, we should have the following
condition:
2
2 1∕2
sin i < (n − n ) . (2.5)
1 2
2 1∕2
2
If (n − n ) > 1, total internal reflection occurs for any incidence angle i. But for most of the practical
1 2
2
2 1∕2
fiber designs, (n − n ) ≪ 1. In this case, as the angle of incidence i increases, decreases and the light
1 2
ray could escape the core–cladding interface without total internal reflection. From Eq. (2.5), the maximum
value of sin i for a ray to be guided is given by
2
2 1∕2
sin i max =(n − n ) . (2.6)
2
1
Therefore, the numerical aperture (NA) of the fiber is defined as
2 1∕2
2
NA = sin i max =(n − n ) , (2.7)
1
2
and i max is called the acceptance angle. Let us define the relative index difference as
n − n 2
1
Δ= . (2.8)
n 1
If the difference between n and n is small, n + n ≈ 2n and Eq. (2.7) can be approximated as
1
2
2
1
1
NA ≈ n (2Δ) 1∕2 . (2.9)
1
Let us construct a cone with the semi-angle being equal to i max , as shown in Fig. 2.5(a). If the incident ray
is within the cone (i < i max ), it will be guided through the fiber. Otherwise, it will escape to the cladding and
then to the jacket, as shown in Fig. 2.5(b). From a practical standpoint, it is desirable to have most of the
source power launched to the fiber, which requires large NA.
Example 2.1
The core and cladding refractive indices of a multi-mode fiber are 1.47 and 1.45, respectively. Find (a) the
numerical aperture, (b) the acceptance angle, and (c) the relative index difference Δ.