Page 81 - Fiber Optic Communications Fund

62 Fiber Optic Communications

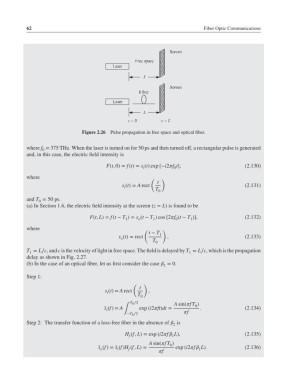
Figure 2.26 Pulse propagation in free space and optical fiber.

where f = 375 THz. When the laser is turned on for 50 ps and then turned off, a rectangular pulse is generated
0
and, in this case, the electric field intensity is

F(t, 0)= f(t)= s (t) exp [−i2f t], (2.130)
i 0
where ( )
t
s (t)= A rect (2.131)
i
T 0
and T = 50 ps.
0
(a) In Section 1.6, the electric field intensity at the screen (z = L) is found to be
F(t, L)= f(t − T )= s (t − T ) cos [2f (t − T )], (2.132)
1 o 1 0 1
where ( )
t − T 1
s (t)= rect , (2.133)
o
T
0
T = L∕c, and c is the velocity of light in free space. The field is delayed by T = L∕c, which is the propagation
1
1
delay as shown in Fig. 2.27.
(b) In the case of an optical fiber, let us first consider the case = 0.
2
Step 1:
( )
t
s (t)= A rect ,
i
T
0
T 0 ∕2 A sin(fT )
̃ s (f)= A exp (i2ft)dt = 0 . (2.134)
i ∫
−T 0 ∕2 f
Step 2: The transfer function of a loss-free fiber in the absence of is
2
H (f, L)= exp (i2f L), (2.135)
f
1
A sin(fT )
0
̃ s (f)= ̃s (f)H (f, L)= exp (i2f L). (2.136)
o i f 1
f