Page 34 - FINAL CFA II SLIDES JUNE 2019 DAY 10

LOS 39.c: Describe and compare how interest rate,
currency, and equity swaps are priced and valued. READING 39: PRICING AND VALUATION OF FORWARD COMMITMENTS
LOS 39.d: Calculate and interpret the no-arbitrage
value of interest rate, currency, and equity swaps. MODULE 39.7: PRICING AND VALUATION OF INTEREST RATE SWAPS

Determining the swap (fixed interest) rate is equivalent to “pricing” the swap. If market interest rates increase during the life of the
swap, the swap will take on a positive value from the perspective of the fixed-rate payer. The value of the swap contract to the
other party (the pay-floating side) will thus be negative.

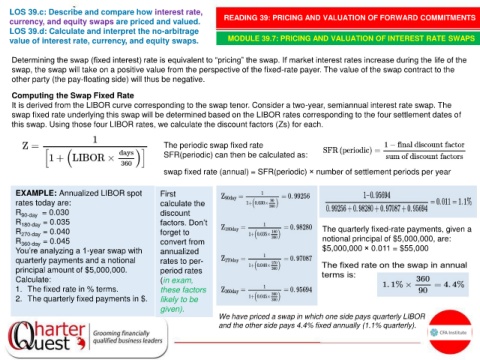
Computing the Swap Fixed Rate
It is derived from the LIBOR curve corresponding to the swap tenor. Consider a two-year, semiannual interest rate swap. The
swap fixed rate underlying this swap will be determined based on the LIBOR rates corresponding to the four settlement dates of
this swap. Using those four LIBOR rates, we calculate the discount factors (Zs) for each.

The periodic swap fixed rate
SFR(periodic) can then be calculated as:

swap fixed rate (annual) = SFR(periodic) × number of settlement periods per year

EXAMPLE: Annualized LIBOR spot First
rates today are: calculate the
R 90-day = 0.030 discount
R 180-day = 0.035 factors. Don’t
R 270-day = 0.040 forget to The quarterly fixed-rate payments, given a
R 360-day = 0.045 convert from notional principal of $5,000,000, are:
You’re analyzing a 1-year swap with annualized $5,000,000 × 0.011 = $55,000
quarterly payments and a notional rates to per-
principal amount of $5,000,000. period rates
Calculate: (in exam,
1. The fixed rate in % terms. these factors
2. The quarterly fixed payments in $. likely to be
given).
We have priced a swap in which one side pays quarterly LIBOR
and the other side pays 4.4% fixed annually (1.1% quarterly).