Page 45 - PowerPoint Presentation
P. 45
LOS 36.c: Describe how the arbitrage-free framework can
be used to value a bond with embedded options. READING 36: VALUATION AND ANALYSIS: BONDS WITH EMBEDDED OPTIONS
LOS 36.f: Calculate the value of a callable or putable bond
from an interest rate tree. MODULE 36.2: VALUING BONDS WITH EMBEDDED OPTIONS, PART 1
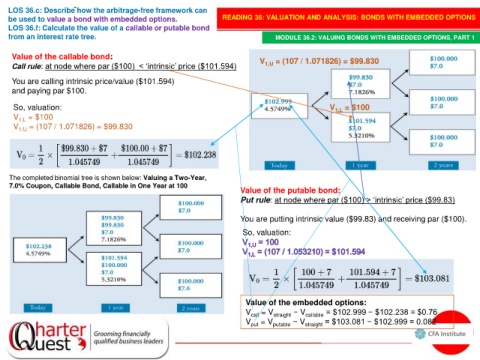
Value of the callable bond: V = (107 / 1.071826) = $99.830
Call rule: at node where par ($100) < ‘intrinsic’ price ($101.594) 1,U
You are calling intrinsic price/value ($101.594)
and paying par $100.
So, valuation: V 1,L = $100
V 1,L = $100
V 1,U = (107 / 1.071826) = $99.830
The completed binomial tree is shown below: Valuing a Two-Year,
7.0% Coupon, Callable Bond, Callable in One Year at 100
Value of the putable bond:
Put rule: at node where par ($100) > ‘intrinsic’ price ($99.83)
You are putting intrinsic value ($99.83) and receiving par ($100).
So, valuation:
Value of the embedded options:
V call = V straight − V callable = $102.999 − $102.238 = $0.76
V put = V putable − V straight = $103.081 − $102.999 = 0.082