Page 26 - FORMULARIO TRIGONOMETRIA - RAIMONDI

Y
1
y = Senx
Senq
q
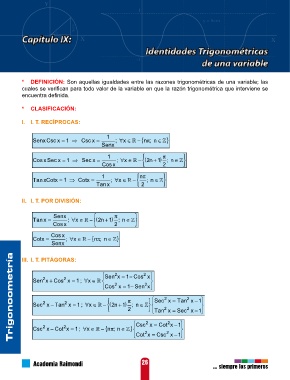
Capítulo IX: X X
Cosq
Identidades Trigonométricas
-1 de una variable

* DEFINICIÓN: Son aquellas igualdades entre las razones trigonométricas de una variable; las
cuales se verifican para todo valor de la variable en que la razón trigonométrica que interviene se
encuentra definida.

* CLASIFICACIÓN:

I. I. T. RECÍPROCAS:

1
SenxCsc x = 1 ⇒ Csc x = ; ∀ x ∈ −{ n ;π n ∈ }

Senx
1 { π }
CosSecx x = 1 ⇒ Sec x = ; ∀ ∈ x − (2 n + ) 1 ; n ∈
Cos x 2
1 { nπ }
Tan xCotx = 1 ⇒ Cotx = ; ∀ ∈ x − ; n ∈
Tan x 2

II. I. T. POR DIVISIÓN:

Senx { π }
Tan x = ; ∀ ∈ x − (2 n + ) 1 ; n ∈
Cos x 2
Cos x

Cotx = ; ∀ x ∈ − n { π ; n ∈ }
Senx
III. I. T. PITÁGORAS:
Trigonoometría Senx + Cos 2 2 2 x = ; x x x −     Cos ; n ∈ }     Sen x        Sec x = Tan x − 

2
2
x 
Senx =− Cos
1
2
1 ∀∈
2
2
x =−
1
{
2
2
π
1 
2
− (
2n + )
; n ∈
Sec x −
Tan x =
1
1
; ∀∈
} 

2
2 2
2
1
Tan x =
Sec x − 

2
1 
2
Csc x =
Cot x − 
2
1

Csc xCot x =
n { π
; ∀∈
−


2
2
1


26
Academia Raimondi   Cotx = Csc x −  ... siempre los primeros