Page 228 - Servo Motors and Industrial Control Theory -

226 Appendix C

engineering values for them. Using final value theorem check that indeed the
two steady errors are zero.
Transform the transfer functions to state space form and calculate the maximum
dynamic position drop when a unit external torque is applied to the load. You
should use numerical integration to find the dynamic position drop. Discuss this
position drop and whether is acceptable for most practical applications. As was
shown in the case for DC servo motors if this dynamic velocity drop is not ac-
ceptable a DC servo motor must be used.

C.4 Electrohydraulic Servo Motors

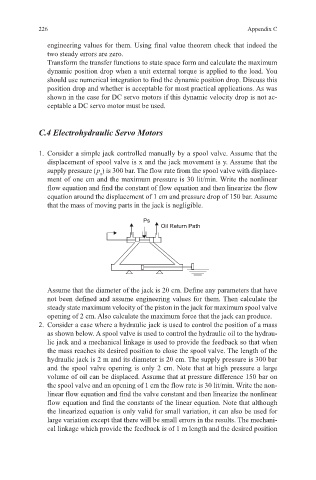
1. Consider a simple jack controlled manually by a spool valve. Assume that the
displacement of spool valve is x and the jack movement is y. Assume that the
supply pressure ( p ) is 300 bar. The flow rate from the spool valve with displace-
s
ment of one cm and the maximum pressure is 30 lit/min. Write the nonlinear
flow equation and find the constant of flow equation and then linearize the flow
equation around the displacement of 1 cm and pressure drop of 150 bar. Assume
that the mass of moving parts in the jack is negligible.

Ps
Oil Return Path

Assume that the diameter of the jack is 20 cm. Define any parameters that have
not been defined and assume engineering values for them. Then calculate the
steady state maximum velocity of the piston in the jack for maximum spool valve
opening of 2 cm. Also calculate the maximum force that the jack can produce.
2. Consider a case where a hydraulic jack is used to control the position of a mass
as shown below. A spool valve is used to control the hydraulic oil to the hydrau-
lic jack and a mechanical linkage is used to provide the feedback so that when
the mass reaches its desired position to close the spool valve. The length of the
hydraulic jack is 2 m and its diameter is 20 cm. The supply pressure is 300 bar
and the spool valve opening is only 2 cm. Note that at high pressure a large
volume of oil can be displaced. Assume that at pressure difference 150 bar on
the spool valve and an opening of 1 cm the flow rate is 30 lit/min. Write the non-
linear flow equation and find the valve constant and then linearize the nonlinear
flow equation and find the constants of the linear equation. Note that although
the linearized equation is only valid for small variation, it can also be used for
large variation except that there will be small errors in the results. The mechani-
cal linkage which provide the feedback is of 1 m length and the desired position