Page 761 - Microeconomics, Fourth Edition

BMappAMathematicalAppendix.qxd 8/17/10 1:10 AM Page 735

A.4 HOW TO FIND A DERIVATIVE 735
( x x 3 x 1 ). Thus, the slope of EF is ¢y/¢x book to learn more about derivatives, including derivatives
( y 3 y 1 )/(x 3 x 1 ). The graph indicates that the slope of of other types of functions not included here.
EF will not exactly measure the slope of the tangent line at
E, but it does give us an approximation of the slope. As the
Derivative of a Constant
graph is drawn, the slope of EF will be less than the slope of
If the dependent variable y is a constant, its derivative with
the line tangent to the function at point E.
We can get a better approximation to the slope at E if respect to x is zero. In other words, suppose y k, where k
is a constant. Then dy/dx 0 .
we choose another point on the graph closer to E, such as
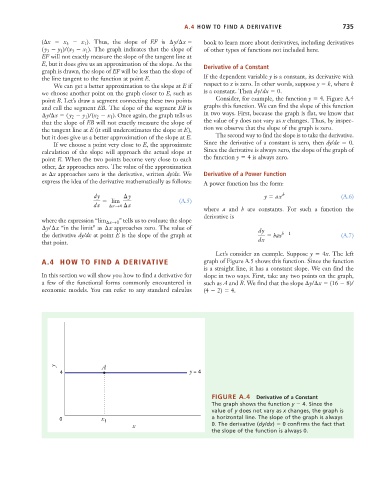
Consider, for example, the function y 4. Figure A.4
point B. Let’s draw a segment connecting these two points
and call the segment EB. The slope of the segment EB is graphs this function. We can find the slope of this function
¢y/¢x ( y 2 y 1 )/(x 2 x 1 ). Once again, the graph tells us in two ways. First, because the graph is flat, we know that
that the slope of EB will not exactly measure the slope of the value of y does not vary as x changes. Thus, by inspec-
the tangent line at E (it still underestimates the slope at E), tion we observe that the slope of the graph is zero.
but it does give us a better approximation of the slope at E. The second way to find the slope is to take the derivative.
If we choose a point very close to E, the approximate Since the derivative of a constant is zero, then dy/dx 0.
calculation of the slope will approach the actual slope at Since the derivative is always zero, the slope of the graph of
point E. When the two points become very close to each the function y 4 is always zero.
other, x approaches zero. The value of the approximation
as x approaches zero is the derivative, written dy dx. We Derivative of a Power Function
express the idea of the derivative mathematically as follows:
A power function has the form:
dy ¢ y y ax b (A.6)
lim (A.5)
dx ¢x S0 ¢x
where a and b are constants. For such a function the
derivative is
” tells us to evaluate the slope
where the expression “lim ¢xS0
¢y/¢x “in the limit” as ¢x approaches zero. The value of dy
the derivative dy/dx at point E is the slope of the graph at bax b 1 (A.7)
that point. dx
Let’s consider an example. Suppose y 4x. The left
A.4 HOW TO FIND A DERIVATIVE graph of Figure A.5 shows this function. Since the function
is a straight line, it has a constant slope. We can find the
In this section we will show you how to find a derivative for slope in two ways. First, take any two points on the graph,
a few of the functional forms commonly encountered in such as A and B. We find that the slope y/ x (16 8)/
economic models. You can refer to any standard calculus (4 2) 4.

y A
4 y = 4

FIGURE A.4 Derivative of a Constant
The graph shows the function y 4. Since the
value of y does not vary as x changes, the graph is
0 x a horizontal line. The slope of the graph is always
1
x 0. The derivative (dy/dx) 0 confirms the fact that
the slope of the function is always 0.