Page 25 - FINAL CFA II SLIDES JUNE 2019 DAY 9

LOS 34.k: Describe modern term structure READING 34: THE TERM STRUCTURE AND
models and how they are used. INTEREST RATE DYNAMICS
MODULE 34.6: INTEREST RATE MODELS

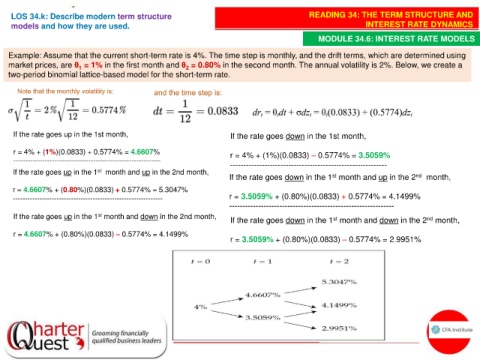
Example: Assume that the current short-term rate is 4%. The time step is monthly, and the drift terms, which are determined using
market prices, are θ = 1% in the first month and θ = 0.80% in the second month. The annual volatility is 2%. Below, we create a
1
2
two-period binomial lattice-based model for the short-term rate.

If the rate goes up in the 1st month, If the rate goes down in the 1st month,

r = 4% + (1%)(0.0833) + 0.5774% = 4.6607% r = 4% + (1%)(0.0833) – 0.5774% = 3.5059%
-------------------------------------------------------------
-----------------------------------------------------------
If the rate goes up in the 1 st month and up in the 2nd month,
st
If the rate goes down in the 1 month and up in the 2 nd month,
r = 4.6607% + (0.80%)(0.0833) + 0.5774% = 5.3047%
-------------------------------------------------------------- r = 3.5059% + (0.80%)(0.0833) + 0.5774% = 4.1499%
--------------------------------------------------------------
If the rate goes up in the 1 month and down in the 2nd month, If the rate goes down in the 1 month and down in the 2 nd month,
st
st
r = 4.6607% + (0.80%)(0.0833) – 0.5774% = 4.1499%
r = 3.5059% + (0.80%)(0.0833) – 0.5774% = 2.9951%