Page 236 - FUNDAMENTALS OF COMPUTER
P. 236
NPP
236 Fundamentals of Computers NPP
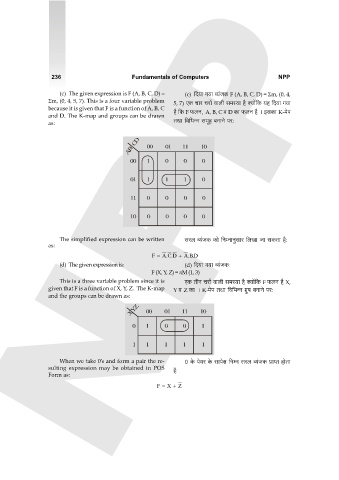
(c) The given expression is F (A, B, C, D) = (c) {X`m J`m ì`§OH$ F (A, B, C, D) = Σm, (0, 4,
Σm, (0, 4, 5, 7). This is a four variable problem 5, 7) EH$ Mma Mam| dmbr g_ñ`m h¡ Š`m|{H$ `h {X`m J`m
because it is given that F is a function of A, B, C
and D. The K-map and groups can be drawn h¡ {H$ F \$bZ, A, B, C d D H$m \$bZ h¡ Ÿ& BgH$m K-_on
as: VWm {d{^ÝZ g_yh ~ZmZo na:
A B C D 00 01 11 10
00 1 0 0 0
01 1 1 1 0
11 0 0 0 0
10 0 0 0 0
The simplified expression can be written gab ì`§OH$ H$mo {ZåZmZwgma {bIm Om gH$Vm h¡:
as:
F = D . C . A + D . B . A
(d) The given expression is: (d) {X`m J`m ì`§OH$:
F (X, Y, Z) = πM (1, 3)
This is a three variable problem since it is EH$ VrZ Mam| dmbr g_ñ`m h¡ Š`m|{H$ F \$bZ h¡ X,
given that F is a function of X, Y, Z. The K-map Y d Z H$m Ÿ& K-_on VWm {d{^ÝZ J«wn ~ZmZo na:
and the groups can be drawn as:
X Y Z 00 01 11 10
0 1 0 0 1
1 1 1 1 1
When we take 0’s and form a pair the re- 0 Ho$ no`a Ho$ gmnoj {ZåZ gab ì`§OH$ àmßV hmoVm
sulting expression may be obtained in POS h¡:
Form as:
F = X + Z