Page 406 - Maxwell House

386 Chapter 8

) | = | | < 1 this progression converges absolutely for
−2 2 2
Since in passive circuits |( 11
11
any discontinuity. Figure 8.1.1d portrays the final closed form expressions.

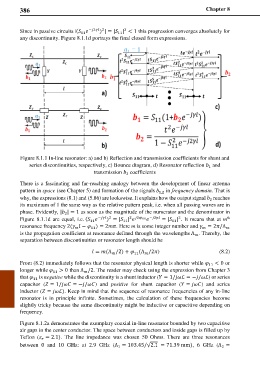
Figure 8.1.1 In-line resonator: a) and b) Reflection and transmission coefficients for shunt and
series discontinuities, respectively, c) Bounce diagram, d) Resonator reflection and
1
transmission coefficients
2
There is a fascinating and far-reaching analogy between the development of linear antenna
pattern in space (see Chapter 5) and formation of the signals in frequency domain. That is
1,2
why, the expressions (8.1) and (5.86) are lookswise. It explains how the output signal reaches
2
its maximum of 1 the same way as the relative pattern peak, i.e. when all passing waves are in
phase. Evidently, | | = 1 as soon as the magnitude of the numerator and the denominator in
2
th
2

Figure 8.1.1d are equal, i.e. ( − 2 11 2 2 11 −2 = | | . It means that at m
) = | |
11
11
resonance frequency 2( − ) = 2. Here m is some integer number and = 2/Λ
11

is the propagation coefficient at resonance defined through the wavelengths Λ . Thereby, the

separation between discontinuities or resonator length should be
= (Λ /2) + (Λ /2) (8.2)

11

From (8.2) immediately follows that the resonator physical length is shorter while 11 < 0 or
longer while 11 > 0 than Λ /2. The reader may check using the expression from Chapter 3

that is negative while the discontinuity is a shunt inductor ( = 1/ℒ = −/ℒ) or series
11
capacitor ( = 1/ = −/) and positive for shunt capacitor ( = ) and series
inductor ( = ℒ). Keep in mind that the sequence of resonance frequencies of any in-line
resonator is in principle infinite. Sometimes, the calculation of these frequencies become
slightly tricky because the same discontinuity might be inductive or capacitive depending on
frequency.
Figure 8.1.2a demonstrates the exemplary coaxial in-line resonator bounded by two capacitive
air gaps in the center conductor. The space between conductors and inside gaps is filled up by
Teflon ( = 2.1). The line impedance was chosen 50 Ohms. There are three resonances

between 0 and 10 GHz: at 2.9 GHz (Λ = 103.45/√2.1 = 71.39 mm), 6 GHz (Λ =
1
2