Page 16 - Bahan Ajar Kalkulus Lanjut

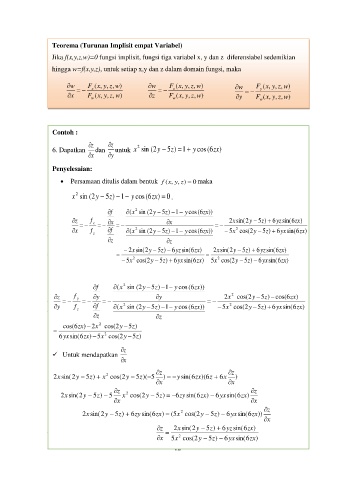
Teorema (Turunan Implisit empat Variabel)

Jika f(x,y,z,w)=0 fungsi implisit, fungsi tiga variabel x, y dan z diferensiabel sedemikian

hingga w=f(x,y,z), untuk setiap x,y dan z dalam domain fungsi, maka

 w = − F x (x , , y , z ) w  w = − F z (x , , y , z ) w  w = − F y (x , , y , z ) w
x  F (x , , y , z ) w z  F (x , , y , z ) w y  F (x , , y , z ) w
w w w

Contoh :

6. Dapatkan z  dan z  untuk x 2 sin 2 ( y − 5z ) = 1 y cos 6 ( zx )
+
x  y 

Penyelesaian:
f
• Persamaan ditulis dalam bentuk (x , y , z ) = 0 maka
x 2 sin 2 ( y − 5z ) − 1− y cos 6 ( zx ) = 0 .

f   (x 2 sin 2 ( y − 5z ) − 1 y cos 6 ( zx ))
−
z  f x x  x  2x sin( 2y − 5z ) + 6yz sin( 6zx )

−
x  = − f z = − f  = −  (x 2 sin 2 ( y − 5z ) − 1 y cos 6 ( zx )) = − − 5x 2 cos( 2y − 5z ) + 6yx sin( 6zx )
z  z 
− 2x sin( 2y − 5z ) − 6yz sin( 6zx ) 2x sin( 2y − 5z ) + 6yz sin( 6zx )
= =
− 5x 2 cos( 2y − 5z ) + 6yx sin( 6zx ) 5x 2 cos( 2y − 5z ) − 6yx sin( 6zx )

−
f   (x 2 sin 2 ( y − 5z ) − 1 y cos 6 ( zx ))
z  f y y  y  2x 2 cos( 2y − 5z ) − cos( 6zx )
= − = − = − = −
y  f z f   (x 2 sin 2 ( y − 5z ) − 1 y cos 6 ( zx )) − 5x 2 cos( 2y − 5z ) + 6yx sin( 6zx )
−
z  z 

cos( 6zx ) − 2x 2 cos( 2y − 5z )
=
6yx sin( 6zx ) − 5x 2 cos( 2y − 5z )
z 
✓ Untuk mendapatkan
x 

z  z 
2x sin( 2y − 5z ) + x 2 cos( 2y − 5z )(− 5 ) = − y sin( 6zx )( 6z + 6x )
x  x 
z  z 
2
2x sin( y − 5z ) − 5 x 2 cos( 2y − 5z ) = − 6zy sin( 6zx ) − 6yx sin( 6zx )
x  x 
z 
2x sin( 2y − 5z ) + 6zy sin( 6zx ) = 5 ( x 2 cos( 2y − 5z ) − 6yx sin( 6zx ))
x 
z  = 2x sin( 2y − 5z ) + 6yz sin( 6zx )
x  5x 2 cos( 2y − 5z ) − 6yx sin( 6zx )
12