Page 245 - Linear Models for the Prediction of Animal Breeding Values 3rd Edition

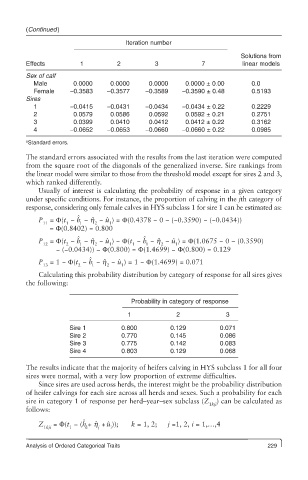
(Continued)
Iteration number

Solutions from
Effects 1 2 3 7 linear models
Sex of calf
Male 0.0000 0.0000 0.0000 0.0000 ± 0.00 0.0
Female −0.3583 −0.3577 −0.3589 −0.3590 ± 0.48 0.5193
Sires
1 −0.0415 −0.0431 −0.0434 −0.0434 ± 0.22 0.2229
2 0.0579 0.0586 0.0592 0.0592 ± 0.21 0.2751
3 0.0399 0.0410 0.0412 0.0412 ± 0.22 0.3162
4 −0.0652 −0.0653 −0.0660 −0.0660 ± 0.22 0.0985
a Standard errors.

The standard errors associated with the results from the last iteration were computed
from the square root of the diagonals of the generalized inverse. Sire rankings from
the linear model were similar to those from the threshold model except for sires 2 and 3,
which ranked differently.
Usually of interest is calculating the probability of response in a given category
under specific conditions. For instance, the proportion of calving in the jth category of
response, considering only female calves in HYS subclass 1 for sire 1 can be estimated as:
ˆ
P = F(t − h − h ˆ − uˆ ) = F(0.4378 − 0 − (−0.3590) − (−0.0434))
11 1 1 2 1
= F(0.8402) = 0.800
ˆ
ˆ
ˆ
ˆ
P = F(t − h − h ˆ − u ) − F(t − h − h ˆ − u ) = F(1.0675 − 0 − (0.3590)
12 2 1 2 1 1 1 2 1
− (−0.0434)) − F(0.800) = F(1.4699) − F(0.800) = 0.129
ˆ
P = 1 − F(t − h − h ˆ − uˆ ) = 1 − F(1.4699) = 0.071
13 2 1 2 1
Calculating this probability distribution by category of response for all sires gives
the following:
Probability in category of response
1 2 3
Sire 1 0.800 0.129 0.071
Sire 2 0.770 0.145 0.086
Sire 3 0.775 0.142 0.083
Sire 4 0.803 0.129 0.068

The results indicate that the majority of heifers calving in HYS subclass 1 for all four
sires were normal, with a very low proportion of extreme difficulties.
Since sires are used across herds, the interest might be the probability distribution
of heifer calvings for each sire across all herds and sexes. Such a probability for each
sire in category 1 of response per herd–year–sex subclass (Z ) can be calculated as
1kji
follows:
ˆ
Z = F(t − (h + h ˆ + ˆu )); k = 1, 2; j =1, 2, i = 1,…,4
1kji 1 k j i
Analysis of Ordered Categorical Traits 229