Page 636 - Microeconomics, Fourth Edition

c15riskandinformation.qxd 8/16/10 11:10 AM Page 610

610 CHAPTER 15 RISK AND INFORMATION

Probability-weighted squared deviation of outcome B 0.40 $0 $0.
2
• Squared deviation of outcome C (payoff of $80) (payoff EV)
2
($80 $100) $400.
Probability-weighted squared deviation of outcome C 0.30 $400 $120.
3. Add up the probability-weighted squared deviations to find the variance:
Variance $120 $0 $120 $240.

If we did the same computation for the investment in a public utility company, with the
probable outcomes shown in Figure 15.3(b), we would find that the variance $80. 1
These results reflect what we can see intuitively by looking at Figure 15.3. The
public utility investment is much less risky than the Internet investment because the
probability that the outcome will equal the expected value (outcome B in both cases)
is 8 in 10 for the public utility investment but only 4 in 10 for the Internet investment.
standard deviation An alternative measure of the riskiness of a lottery is the standard deviation,
The square root of the which is simply the square root of the variance. Thus, the standard deviation of the
variance. Internet stock is 1240 15.5, and the standard deviation of the public utility stock
is 180 8.9.
If the variance of one lottery is bigger than the variance of another lottery, it fol-
lows that the standard deviation of the first lottery will be bigger than the standard de-
viation of the second lottery. Thus, the standard deviation provides us with the same
information about the relative riskiness of lotteries as does the variance.

APPLICA TION 15.1
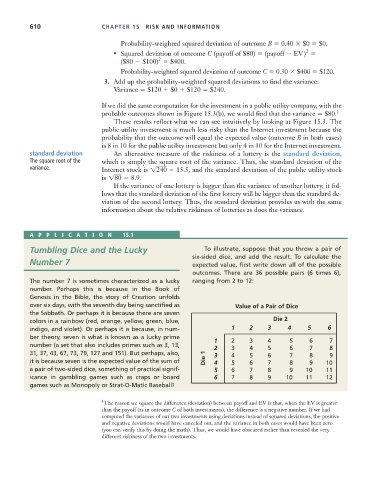
Tumbling Dice and the Lucky To illustrate, suppose that you throw a pair of
six-sided dice, and add the result. To calculate the
Number 7 expected value, first write down all of the possible
outcomes. There are 36 possible pairs (6 times 6),
The number 7 is sometimes characterized as a lucky ranging from 2 to 12:
number. Perhaps this is because in the Book of
Genesis in the Bible, the story of Creation unfolds
over six days, with the seventh day being sanctified as Value of a Pair of Dice
the Sabbath. Or perhaps it is because there are seven
colors in a rainbow (red, orange, yellow, green, blue, Die 2
indigo, and violet). Or perhaps it is because, in num- 1 2 3 4 5 6
ber theory, seven is what is known as a lucky prime
1 2 3 4 5 6 7
number (a set that also includes primes such as 3, 13,
2 3 4 5 6 7 8
31, 37, 43, 67, 73, 79, 127 and 151). But perhaps, also, 3 4 5 6 7 8 9
it is because seven is the expected value of the sum of Die 1 4 5 6 7 8 9 10
a pair of two-sided dice, something of practical signif- 5 6 7 8 9 10 11
icance in gambling games such as craps or board 6 7 8 9 10 11 12
games such as Monopoly or Strat-O-Matic Baseball!

1 The reason we square the difference (deviation) between payoff and EV is that, when the EV is greater
than the payoff (as in outcome C of both investments), the difference is a negative number. If we had
computed the variances of our two investments using deviations instead of squared deviations, the positive
and negative deviations would have canceled out, and the variance in both cases would have been zero
(you can verify this by doing the math). Thus, we would have obscured rather than revealed the very
different riskiness of the two investments.