Page 82 - BUKU ARA

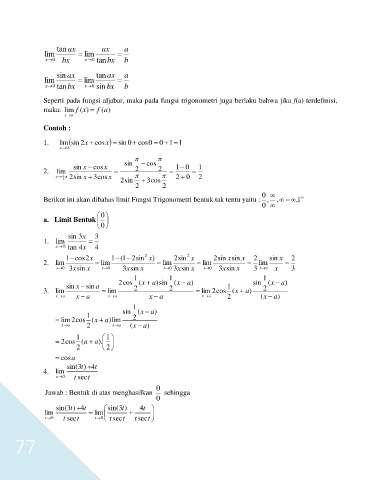
tan ax ax a
lim  lim 
x0 bx x0 tan bx b
sin ax tan ax a
lim  lim 
x0 tan bx x0 sin bx b

Seperti pada fungsi aljabar, maka pada fungsi trigonometri juga berlaku bahwa jika f(a) terdefinisi,
maka: lim ( )f x  f ( )
a
x a
Contoh :
1. lim sin 2  cosx  sin  0 cos 0  0  1 1
x
x  0
 
sin  cos x sin  cos 1 0 1
x
2. lim  2 2  
x
1
x   2 sin  3 cos x 2 sin   3 cos  2  0 2
2
2 2
0 

,
Berikut ini akan dibahas limit Fungsi Trigonometri bentuk tak tentu yaitu : , ,    1
0 
 0 
a. Limit Bentuk  
 0 
sin 3x 3
1. lim 
x  0 tan 4x 4
1 cos 2x 1 1 (  2 sin 2 ) x 2 sin x 2 sin x sin x 2 sin x 2
2
2. lim  lim  lim  lim  lim 
x  0 3x sin x x  0 3x sin x x  0 3x sin x x  0 3x sin x 3 x o x 3
1 1 1
sin x  sin a 2 cos 2 (x  ) a sin 2 (x  ) a 1 sin 2 (x  ) a
3. lim  lim  lim 2 cos (x  ) a
x a x  a x a x  a x a 2 (x  ) a
1
sin x (  a)
1
 lim 2 cos x (  a lim 2
)
x a 2 x a x (  a)
1  1 
 2 cos a (  a). 
2  2 
 cos a
sin( 3 t)  t 4
4. lim
x 0 t sec t
0
Jawab : Bentuk di atas menghasilkan sehingga
0
4
sin( 3 t)  t  sin( 3 t) t 4 
lim  lim   
t 0 t sec t t 0  t sec t t sec  t

77