Page 100 - BUKU ARA

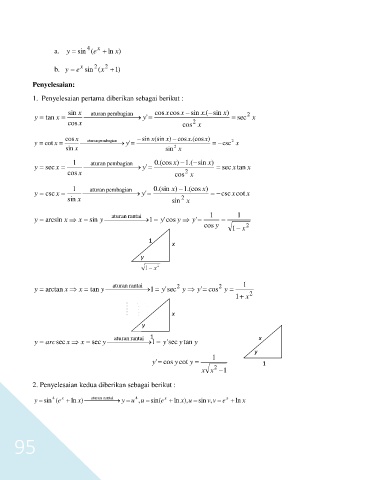
x
a. y  sin 4 (e  ln ) x

b.  ey x sin 2 (x 2  ) 1

Penyelesaian:
1. Penyelesaian pertama diberikan sebagai berikut :


sin x aturan pembagian cos xcos x sin x.( sin x) 2
y  tan x         y'  sec x
cos x cos 2 x

cos x  sin x(sin x)  cos x.(cos x)
y  cot x   aturan pembagian y'   csc 2 x





sin x sin 2 x
1 aturan pembagian 0 .(cos x)  1 .( sin x)
y  sec x         y'  sec x tan x
cos x cos 2 x
1 aturan pembagian 0 .(sin x)  1 .(cos x)
y  csc x         y'   csc xcot x
sin x sin 2 x

rantai


y  arcsin x  x  sin y  aturan   1  ' y cos y  ' y  1  1

cos y 1 x 2

1 x
y

rantai
2
2


y  arctan x  x  tan y  aturan   1  ' y sec y  ' y  cos y  1


1 x 2
x
y
rantai

1 

y  arcsec x  x  sec y  aturan   1 y sec y tan y x

'
y
1
'y cos y cot  1
y
x x 2  1
2. Penyelesaian kedua diberikan sebagai berikut :
4
x
x







y sin 4 e ( x  ln x)  aturan rantai y  u , u sin( e  ln x), u sin v, v  e  ln x
95